Benoit Mandelbrot, Fractal Geometer Extraordinaire
Benoit Mandelbrot died on Thursday Oct. 14, 2010, at the age of 85. This is the end of an era.
I’m not sure I can adequately put into words what Mandelbrot’s discoveries and work mean to me, and the effect they have had on my life.
Let’s start with the name of my blog and website: Strange Attractor. I’ve been meaning to write a post about what a strange attractor is. I even have my own haiku definition of a strange attractor:
Eavestroughs of chaos
Funnelling beauty into
Following strange paths
A strange attractor is a concept from chaos theory and nonlinear mathematics. Some strange attractors are fractals. That’s probably all you need to know for this post.
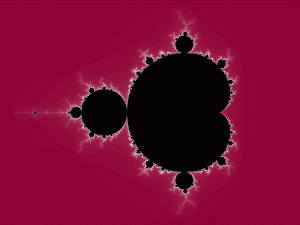
Benoit Mandelbrot did groundbreaking work on fractal geometry. He coined the term “fractal geometry”. The Mandelbrot Set, which the most famous and recognizable fractal in the world, is named after him because he was one of the first people to run a computer simulation to see what it looks like, and he was the first one to study it in detail. He is one of the key people who, building on the scattered mathematical oddities of the past, formed the fields of study we now call chaos theory, fractal geometry, and nonlinear science.
I was introduced to fractals by my high school math teacher, Darren Luoma, and it changed my life.
I never looked at a tree the same way again. I had new ways of looking at art, new ways of recognizing patterns. The structure of imagined things and real things in the world unfurled in my mind in new configurations. I had a sudden feeling for how something so tiny as DNA could encode something so complex as life.
A dissatisfaction I had had with the oversimplification of the world into the clumsy abstractions I’d been taught was suddenly alleviated. (At least some of the dissatisfaction regarding geometrical relationships in nature was alleviated. This type of dissatisfaction about the limitations of abstractions on a variety of topics is still very much with me.) There were now new abstractions that explained things better, that gave me different and fascinating ways to understand the world. It was like a space opened up inside of me. It was like my mind untangled and grew.
The following quote is a famous one, and once I understood the fractal models of the structures of clouds, mountains, coastlines, bark and lightning, there was no going back to the previous simplifications.
“Clouds are not spheres, mountains are not cones, coastlines are not circles, and bark is not smooth, nor does lightning travel in a straight line.” — Benoit Mandelbrot, The Fractal Geometry of Nature, 1983.
It was the indignation and dissatisfaction I had been feeling, expressed. Clouds are not spheres! No they’re not! You can model them as spheres, but you lose a lot of what makes them interesting if you do so. More importantly, a sphere is not the only available tool, nor is it the best tool, for modelling them. What this quote brought me was vindication and a doorway to something better. The ideas it referred to let me escape from the monopoly on my imagination of the previous model I’d been spoon-fed.
If I ever have children, if I teach them to draw trees, the lesson will start with a simple bifurcating algorithm. They’ll probably pick up the other model, a circle or triangle with a line, from their teachers or peers. It’s not that the other model is bad. It’s actually quite useful in a variety of situations. But it’s not enough for me. And if it’s the only model you have in your imagination of a tree, you can end up thinking that that’s all there is to a tree, without even realizing the abstraction that just took place, or what you’re missing out on. It’s like the problem of imposing legibility, that is, making a map, then forgetting the territory, then attempting to change the territory to fit the map and wondering why it doesn’t work, and leaving carnage in the wake. Ok, not necessarily the carnage part. But usually something gets lost in the process of doing something like that, even if it is no more nor less than the flexibility of a mind.
The Mandelbrot Set is quite beautiful. I had a visceral, aesthetic response to it. By exploring it, I got more than abstractions, I got a feeling for them. I got experience. There are some things you can know abstractly that are different when you know them from experience. I spent hours delving into deep parts of the Mandelbrot Set, and then I’d come back for more. The wonderful thing is, there was always more. That’s what infinity means. 🙂
The Mandelbrot-Julia set was the first 4-dimensional object that I attempted to visualize. There was a setting in the computer program I was using, Fractint, that displayed one 3-dimensional slice at a time. I moved through it, slice by slice, thinking about how to put it together in my head.
Fractint, by the way, was made by the Stone Soup Group, which was named after the story of the stone soup. In retrospect, that was my first introduction to the ideas of software collaboration and freedom, which have become important to me.
I could go on with more examples and stories about what Mandelbrot’s work, and fractals in general, mean to me. I think I’ll stop now. I feel honoured to have benefited from his ideas, and to have lived at the same time as him. I feel sad today, but also I feel like saluting him. Goodbye Benoit Mandelbrot, and thank you.
Related links:
New York Times Obituary of Benoit Mandelbrot
Benoît Mandelbrot’s Wikipedia Page
Fractint’s Wikipedia Page
Mandelbrot Google Image Search
The Most Famous Fractal: The Mandelbrot Set (PBS Nova)

[…] This post was mentioned on Twitter by Darcy Casselman and Darcy Casselman, strangeattractor. strangeattractor said: Blog Post: Benoit Mandelbrot, Fractal Geometer Extraordinaire http://www.strangeattractor.ca/wp/2010/10/16/benoit-mandelbrot/ […]
I am new to fractals, and non-mathematical, but the beauty and logic have captured and captivated me.
If fractals are everywhere, and they repeat into infinity, can this explain the universe? Or rather, explain why the universe can not be explained? Can it explain that everything that is called the universe has always been out there, and always will be, and only parts (worlds, people, things) can be destroyed, only to be recreated?
If everything is repeated and repeated, are there other worlds out there like ours, repeated and repeated?
Lenore, when we see fractal-like structures in nature, they often don’t repeat into infinity. There is often some level at which the iterations stop. For example, a tree doesn’t keep branching forever, it has a smallest branch. One of the things that has helped my understanding of why they stop repeating is Adrian Bejan’s work on constructal theory. Basically, at different scales the optimal way to do things changes, so the iterations stop at the level at which it becomes more efficient to use a different process or structure.
That said, the using the idea of fractals metaphorically, and experiencing the vastness of the Mandelbrot set by exploring it with computer programs, has given me an idea of how simple rules and a small amount of information can result in complex results. For example, the idea that something as small as DNA could lead to something as complex as a lifeform is a little bit more comprehensible to me, though still quite mysterious.
As for the idea of the universe being recreated, I think Sir Roger Penrose has done some work on what might have happened before the Big Bang, and it looks like, from preliminary results, that there are echoes of another universe.